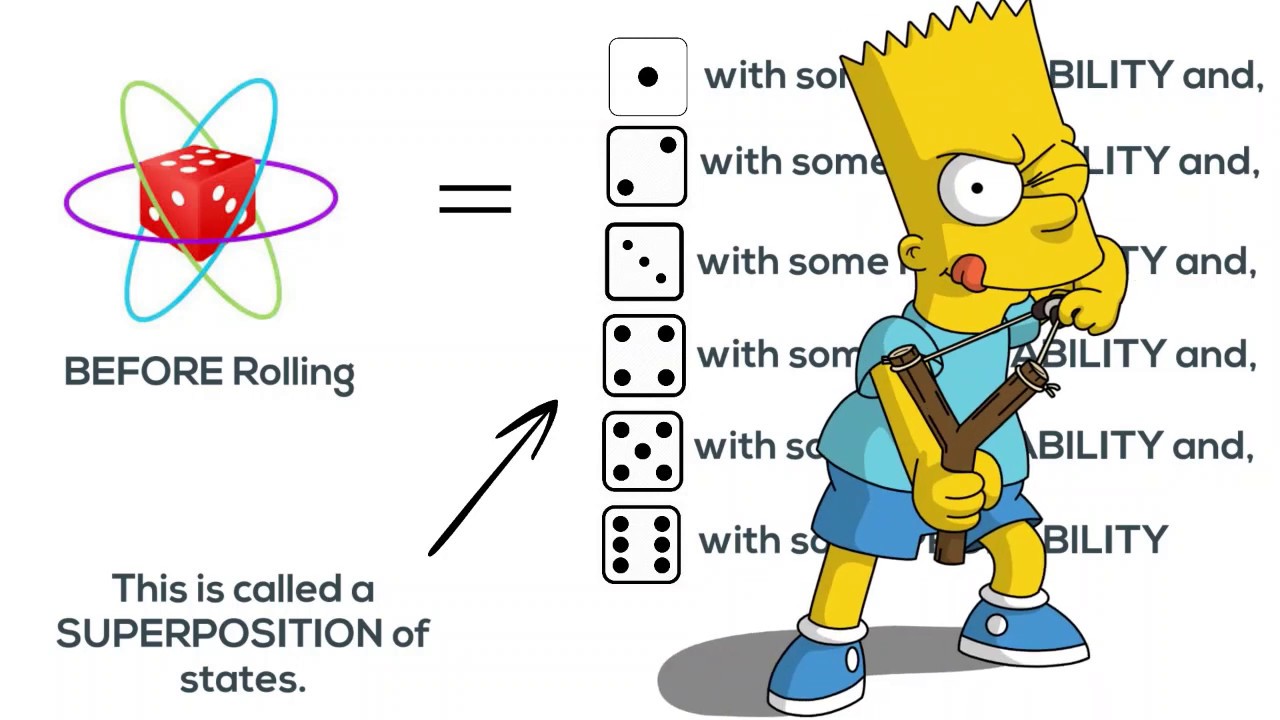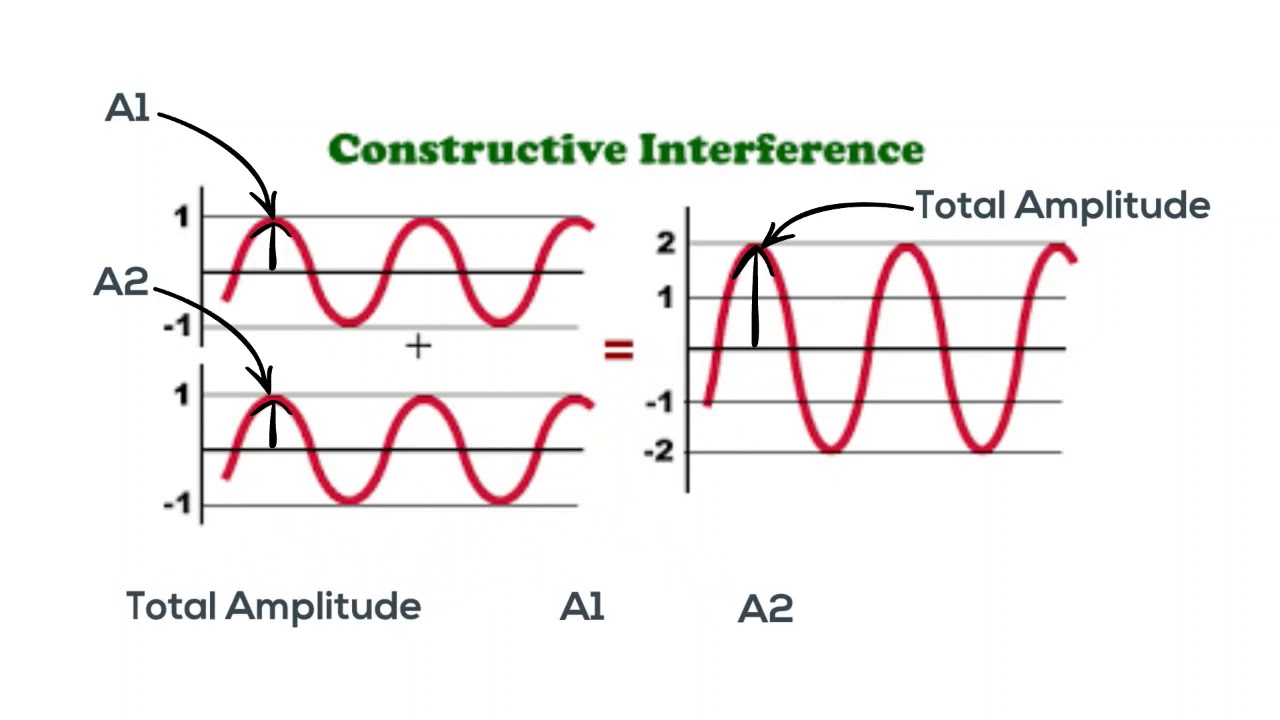
WELCOME TO THE PARTICLE EXPERIENCE

In order to tackle the massive problem of studying everything in the entire universe, Physicists apply the philosophy of reductionism. This leads to the Standard Model, the penultimate theory of the 20th century, consisting of the most fundamental particles and interactions from which all other systems in the universe are born. Script: Physics is the study of the universe – which is tantamount to saying Physics is the study of EVERYTHING. Needless to say, studying everything can be quite the intimidating task. But physicists are clever. They realized that the study of everything could really be boiled down to the study of the few fundamental ingredients which go into building everything else. (Which begs the question: What does it mean for something to be fundamental? To a physicist, fundamental is synonymous with indivisible. A fundamental particle is one which cannot be broken down to any parts beyond itself.) Take, for example, a star in the night sky. Like others before them, physicists wondered what they were and came to realize that – contrary to popular belief—they were not diamonds in the sky, but rather a hot, luminous gas. Zoom in and you’ll see that each star is in fact comprised of chemical compounds called molecules, which are themselves composed out of atoms of the elements of the periodic table – in the case of a star primarily hydrogen and helium. Zoom in even further and you’ll find that each atom of an element is itself formed out of a hard nucleus containing protons and neutrons surrounded by a cloud of orbiting electrons. For a long time, scientists believed that these were the fundamental constituents of all matter – protons, neutrons and electrons. Upon even further magnifications, however, it has been realized that, while electrons constitute indivisible, fundamental particles, protons and neutrons are in fact made up of even smaller constituent particles called Quarks. A proton contains two up quarks and one down quark, while a neutron contains one up quark and two down quarks. A particle composed of two or more quarks, like a proton or a neutron, is called a hadron. Remarkably, there are even still more fundamental particles which go into forming the stars we see in the night sky. Particles called gluons hold quarks together inside hadrons through an interaction called the Strong Nuclear Force. Over time, as a star ages and exhausts its resources of hydrogen and helium it begins to decay radioactively through an interaction called the Weak Nuclear force facilitated by particles known as the W and Z Bosons. In fact, the only reason we can see stars to begin with is because they emit particles of light called photons which are released as byproducts of the electromagnetic interactions occurring between charges in the star. When all is said and done Physicists have determined that there are 17 indivisible, fundamental particles which are responsible for producing (almost) all the matter we observe in space and facilitating (almost) all the interactions between matter through the three fundamental forces: Electromagnetic, Strong and Weak. Those are a couple of big “almost”, but that is for another video! These 17 particles form what is called the Standard Model of Particle Physics, which, despite its shortcomings, is quite handedly the most complete, all encompassing model for understanding the physical world (currently accepted by the vast majority of practicing physicists (sorry String Theory!).) Miraculously, physicists can understand the physical properties and interactions of any system cooked from a recipe of standard model ingredients simply by understanding the properties and interactions of the underlying particles. Thus, we went from having to worry about EVERYTHING to having to worry about 17 different things. Not a bad magic trick, huh?

The Standard Model consists of 17 particles and three fundamental interactions. In this video we give a quick run through how these particles are described, and how they make physics happen. Script: As the action of a play arises from the relationships between the different characters and their environment, physical processes in the universe arise out of the interaction between fundamental particles and space. Which is to say: if the Universe were a play, the fundamental particles of the standard model would be the cast of characters with spacetime as the stage. At a high level the Standard Model can be broken down into two major categories of character – Fermions and Bosons. The fermions are kind of like the main characters in the play, while the bosons are something like supporting characters. More on this in just a bit. The fermions are further separated into what are called “Generations” of matter depending upon their mass. We might think of this distinction as determining the level of importance of a particular fermion character since the mass of a particle determines its level of stability and in turn its average lifetime. Hence the first generation of matter are the primary main characters, the second the secondary, and the third the tertiary. Each particle, fermion and boson alike, is associated with a list of parameters, called quantum numbers, which outline how it will react in the presence of other particles. This is analogous to how the attributes, attitudes and aspirations of different people impact the way they relate to others. Some of these numbers – like charge and mass – are probably quite familiar. Others – like spin, color, or flavor – are probably not. The action of particle physics happens through what the physicists like to call: Interactions. It turns out that every quantum number is associated with one of these interactions. For instance, a particle’s charge and spin determine how it interacts electromagnetically. Color and flavor determine how it interacts with the Strong and Weak nuclear forces respectively. Finally, a fundamental particle’s mass is a representation of its interaction with the Higgs Field. The actual business of how fundamental particles interact with one another is surprisingly relatable. Each interaction is facilitated by what is called a “messenger” boson – hence why I referred to bosons as supporting characters-- which travels (virtually) between partners of interacting particles communicating their respective quantum numbers. Think of the messenger boson as being like your friend in middle school who told the girl you liked that you liked her. Upon receiving the message, she would either come and talk to you, or stay as far away from you as she possibly could – depending on what she thought of the key elements of your personality. Similarly, when two particle “see” each other, a messenger boson will come along to relate the other’s quantum numbers. If these particle’s quantum numbers are complementary – for instance if the particles have opposite charges – they will be drawn closer together. If the numbers are not complementary – for instance if the particles have like charges – they will be repelled from one another. Bosons, in addition to carrying the interaction mail between fermions, can also interact on their own accord with the details of their interaction being carried out by still other bosons. When all is said and done, every physical occurrence we observe in nature can be traced back to the 17 particles of the Standard Model and the interactions brought about by their quantum numbers… that is everything except Dark Matter, and Dark Energy, oh, yeah, and Gravity! Which is to say, if the Universe were a play, the fundamental particles of the standard model were its characters we might not want to get too comfortable, new cast members are almost certainly on the way!

In this video we zoom out from the world of particle physics to discuss an area of study known as Classical Mechanics. In this more familiar realm of physics we discover the mystical incantation known as the Principle of Least Action which acts as the Physicist's crystal ball. Script: In our first series of videos we laid out the basics of the Standard Model. Broadly speaking, the Standard Model represents the most fundamental particles with which all other matter is composed, and the facilities for interaction between such particles through the intermediaries of three of the fundamental forces of Nature: Electromagnetic, Strong and Weak. Now, we want to discuss how Physicists utilize the Standard Model to make predictions about the universe. The realm within which the Standard Model is applied is called Quantum Field Theory. Before we get to there, however, it will be helpful to consider a more familiar area of Physics known as Classical Mechanics. Classical mechanics involves the analysis of physical systems which are on the length and energy scales we are used to living in. For this reason, the complexities of Quantum Mechanics associated with the dynamics of individual particles are essentially negligible – they’re simply so small compared to the system we are analyzing to be important. Ultimately, Classical Mechanics is only an approximation. The true physics of every situation must be described quantum mechanically, because everything in nature is composed of the fundamental particles of the Standard Model. But as it stands, Classical Mechanics is a really good approximation. Good enough for engineers when they design buildings and bridges, and even good enough to send men to the moon! Most importantly, the simplicity of Classical Mechanics makes it the perfect Segway into discussing the way Physicists go about making predictions. All of classical mechanics can be encapsulated in a single question: Given information about where a physical system is and where it is going at a given time, predict where the system will be and where it will be going at all later times. To the uninitiated this sounds a lot like fortune telling, and, to a certain extent it is. But there’s an important difference between a theoretical physicist and your local clairvoyant: The physicist is totally transparent about where his predictions come from, and in the world of classical mechanics that means the Principle of Least Action. To get straight down to brass tacks, Nature is lazy. Or, to be kinder, Nature is efficient. Whenever any system in nature needs to decide what it will do next, it chooses the option that minimizes this thing I called the action. So, what exactly is action, then? As you might have guessed, action is related to energy. On some level we can think of it as being the total energy expenditure to go from one state of being to another. Strictly speaking, the action is given by an integral, or sum, over a mathematical functional known as the Lagrangian. The Lagrangian turns out to be perhaps the most important object of analysis in all of Physics. Simply put, the Lagrangian of a given system is equal to the difference between the system’s kinetic energy – that is the energy associated with the motion of the system – and the system’s potential energy – that is the energy associated with the forcefield acting on the system due to the fundamental interactions and Gravity.

In this video we get better acquainted with the Principle of Least Action and the Lagrangian which we've heard so much about. Script: It’s easy to get caught up in all these definitions, but truthfully the principle of least action and the role of the Lagrangian are both aspects of nature which are very familiar to the way we as human beings make our own decisions. Specifically, the Principle of Least Action is Nature’s rule for how to travel, and the Lagrangian is Nature’s GPS. Let me explain. Imagine you’ve decided to go to your favorite restaurant. The first question I’ll ask you is this: how would you like to get there? Unless you’re really into taking the scenic route through life, more likely than not your answer will be something like: “as fast as possible”. Translation: when we travel from point A to point B, we seek to do so in the least amount of time possible. Double translation, when we move, we do so according to what might be called a Principle of Least Time. Which is to say, time is to us what action is to nature. The Second Question I’ll ask you is: how will you make sure you get from point A to point B in the least time possible? To which you should probably respond, I’ll just punch the address into my GPS. Now, you might contest, “but I know the best path to my favorite restaurant, I’ve driven it a million times”. Only, when trying to determine the best way to drive from your home to the restaurant there’s more than one thing you need to take account of. In particular, you need to determine what is the most direct path given the available streets, and what path is least affected by traffic at the time of your journey – the latter of which you couldn’t possibly be aware of up to the minute without a GPS. These two considerations map perfectly on to the two parts of the Lagrangian. The streets are the kinetic energy, the traffic is the underlying potential. In the absence of any external force (if the potential energy is zero) the path of least action is just a straight line, just like how in the absence of traffic the best path to your restaurant is just the one that is the most direct. However, when forces are at play, the path of least action becomes curved. This is analogous to how traffic can force you to take a detour from your direct path, saving precious time in the long run. It is a remarkable fact that essentially every question which can be asked in the study of classical mechanics can be answered simply by applying the principle of least action. However, I’ll end the video by telling you that I have some good news and some bad news. First, the bad news: where we’re going – the world of Quantum Mechanics – the principle of least action is no longer capable of solving all of our problems. The good news is, the action and the Lagrangian, albeit applied in a different way, will still be enough to find our answer!

Things get tricky when we zoom back down to the world of fundamental particles. In quantum mechanics, nothing can be taken for granted. Script: The Principle of Least Action was so successful in solving the problems of Classical Mechanics that many Physicists of the late 1800s and early 1900s were ready to call the subject a closed book. It was just at this time that a young aspiring scientist by the name of Max Planck expressed his desire to enter a graduate program in the study of Physics in Germany. He was flatly told, "it would be better to look elsewhere, for physics was at the end of the road, with so little really worthwhile left to do." Undeterred, Planck pressed onward. Quite to the contrary of what he was told, Planck would go on to become one of the founders of what we now call Quantum Mechanics – and Physics has never been the same. What sets Quantum Mechanics apart from Classical Mechanics is quite simply that Quantum Mechanics is fundamentally random. Even if he is given all the information that can possibly be known about a quantum system, a physicist cannot, with absolute certainty, predict what the system will do next. He can only predict the probabilities that the system will do any of the numerous things it possibly could do. Most of us are familiar with probability from our experience with games of chance. For example, we know that when we roll a fair die – that is a cube shaped die with equally sized sides-- there is a one in six chance that it will land on any of its given faces. As abnormal as it may sound, we might think of any quantum system as being like a kind of die, only it could have many more than six faces—corresponding to the different “states” the system could be in, and each face can have a different size—proportionate to the likelihood a given state will be realized. From this perspective, the job of the physicist in analyzing a quantum system is simply to determine the makeup of the system’s quantum dice. If the idea of nature being fundamentally random makes you feel uncomfortable, don’t worry – you’re not alone. Einstein himself was famously opposed to the probabilistic nature of Quantum Mechanics, even going so far as to say, “God does not play dice with the universe.” However, no experiment has ever been successful in disproving the predictions of Quantum Mechanics, and believe me, many have tried. Like it or not, Quantum Mechanics and all its randomness is here to stay. So, now that we know what our job is, and we’ve accepted that there’s no way around it, it’s time to get down to business. Still, it may feel like we’ve been dropped in a strange new world with no signposts or indication of where to go looking for our answer. But, if there is one guiding principle throughout Physics it is: when in doubt look to the action. So, that is just what we’ll do.

With some help from Bart Simpson, we get introduced to one of the most fundamental problem solving tools in the physicist's toolbox. I hope you like being in every possible state at once! Script: We left off pondering the following question: How can we determine the probability that a quantum system in some initial state, I, will transition into some final state, F? It turns out, the answer to this question is surprisingly straightforward, at least conceptually. Because a quantum system cannot be in a determined state before it is measured, we are forced to say that the system is in a superposition of all its possible states at any given time. To understand what this means, think of a badly-behaved child sent to his room to go to sleep. He is so unpredictable, that once his door is closed you couldn’t say with any certainty where he’s going to be – in principle he could be anywhere in the room (except, perhaps, in his bed!). It is only when you open the door again and check on him that his state of being becomes clear. Since a quantum system occupies all its possible states between measurements, it stands to reason that the probability the system will transition from one state of being to another state of being should be something like a sum over all the different ways this state transition could be achieved. For instance, the probability our child is on top of his dresser is something like the probability he jumped to it from his bed, plus the probability he climbed the drawers like a ladder, plus the probability he knocked it down to be able to sit atop it and so on over all of the different possible ways he could think to get up there. In fact, this is exactly the recipe a Physicist follows to answer our question, only, because Physicists like to be fancy, we give the “sum over all the different ways” the complicated sounding name: Path Integral. At its core, the Path Integral formulation of Quantum Mechanics is really no more complicated than the sum we did in trying to predict where our child would be.

We finally put everything together and see what role the action plays in Quantum Physics. Along the way we are forced to deal with a tricky fact about probabilities in quantum mechanics.

The culmination of this introduction to particle physics! Having triumphed over the daunting path integral, we are now introduced to a formalism which allows us to solve the same problem but simply by drawing a pretty picture. Go figure... Script (shortened due to video description constraints): Putting together our puzzle pieces, we can construct a very simple recipe for answering this question: Define the initial state (I) and final state (F) of interest by identifying the Standard Model particles present in each state. Indicate the trajectories of each particle to establish the Kinetic Energy associated with each state. Determine all the possible ways these particles could interact with each other through the Standard Model Forces on the way from State I to State F. Associated with each interaction is a Potential Energy and a messenger Standard Model particle connecting the initial and final state. Using the Kinetic Energy associated with the particle trajectories, and the Potential Energy associated with the particle interactions, form the Lagrangian (Kinetic Energy – Potential Energy) of each possible transition mode. From the Lagrangian, calculate the Action required to make each transition which is directly proportionate to the Phase of the Probability Wave associated with the transition possibility. Compute the Path Integral by taking the sum over probability waves associated with each transition. Since effectively every physical system is composed from some combination of Standard Model Particles, which interact via the Standard Model Forces – this recipe constitutes a method for analyzing effectively every physical system in nature. But it gets even better. The great Richard Feynman – to who we owe the Path Integral Formulation of Quantum Mechanics, among his many contributions to the field of Theoretical Physics – developed an ingenious technique for representing our recipe with nothing more than a few pictures. This technique is now called the Feynman Diagram and is one of the most widely used methods for communicating Physics. Simply put, the Feynman Diagram is comprised of Four Parts The canvas upon which the Feynman Diagram is drawn represents a coordinate system, with the x-axis typically denoting space and the y-axis typically denoting time. At the bottom of the diagram, corresponding to the beginning of time, we draw the initial state of the system, and at the top of the diagram, corresponding to the end of time, we draw the final state of the system. The trajectory of the particles is then depicted through straight lines – which encapsulate the Kinetic Energy of the System. Where these straight lines meet, we think of the particles as interacting. These points are called the “vertices”. Associated with each vertex is an interaction Potential Energy. Finally, the system is brought from the initial state to the final state by the intermediary of a “messenger” particle which facilitates the interaction. The messenger particle is denoted by its own line. Once a Feynman Diagram is drawn, it can be translated directly into a Path Integral using what are called the Feynman Rules. Strictly speaking, these rules tell us how to form the initial and final particle Quantum States, the interaction Lagrangian and the Messenger Particle Operator, which come together to form the Path Integral. Intuitively, however, we can understand this procedure by recognizing each piece of the Feynman Diagram as relaying a mathematical object, and the product of these objects results in the Path Integral. Thus, the pieces of our puzzle quite literally come together to form the Feynman Diagram, which constitutes a symbolic solution to our ultimate question. It is a triumph of epic proportions to be sure. Quantum Field Theory – the edifice within which the Feynman Diagram is applied – is quite rightly considered the most accurate and successful theory in all of science. However, to close I must warn that, despite how it might seem, the puzzle is not complete – the Standard Model, for all its success, is riddled with missing pieces. Now, with our foundation set, we are prepared to entered the great unknown and confront the riddles of contemporary Physics head on!